Publications

 
  Complete list of publications (last updated 2023/July/12):

 

A list of citations can be found here.

Edited books:

 
1. G- Hernandez-Duenas & M. A. Moreles. Mathematical and Computational Models of Flows and Waves in Geophysics. Springer (Book) (1), pp 1-195 (2022).
https://doi.org/10.1007/978-3-031-12007-7
 
 
 
 
 
 
Figure: Book about Geophysical applications.  
 
 

Published papers:

22. Weakly compressible two-layer shallow water flows along channels.
S. Shah & G. Hernández-Dueñas.
Journal of Scientific Computing (2024)
Link to Journal

 

 

 

 

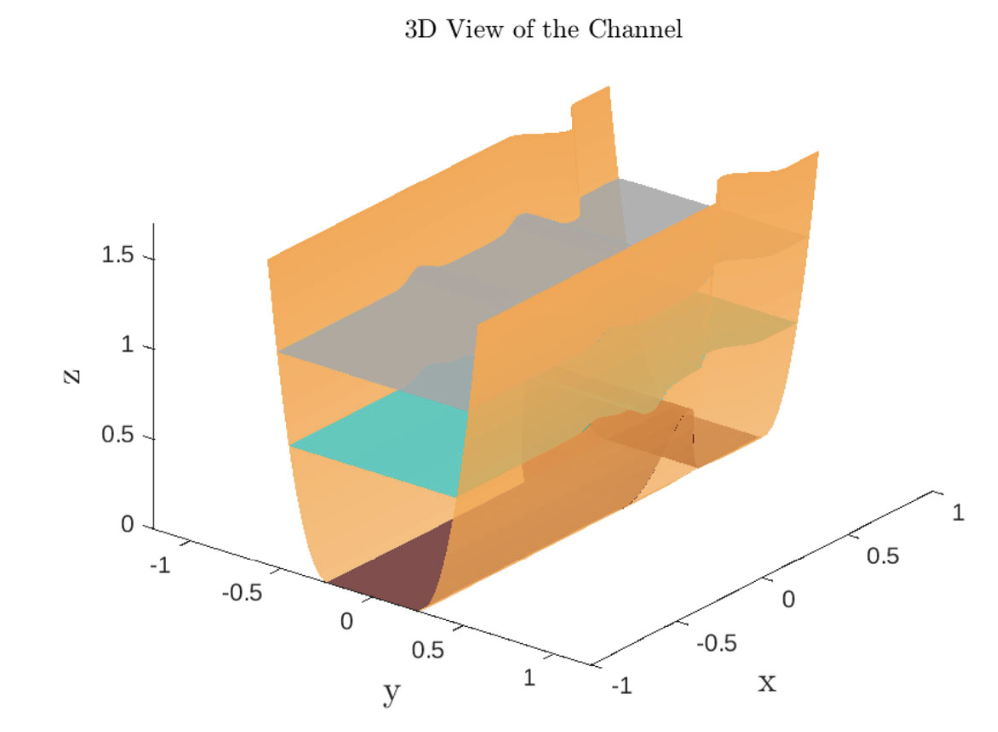
Figure: Two-layer flow in channels.

 
21. Exploring the excess of cloud condensation nuclei and rain suppression using a minimal three-dimensional Boussinesq model with bulk cloud microphysics.
O. Guerrero-Medina & G. Hernández-Dueñas.
Physics of Fluids (2024)
Link to Journal

 

 

 

 

Figure: Graph of aerosols & rain.

20. Global well-posedness of a model for precipitating convection with hydrostatic pressure under fast autoconversion and rain evaporation conditions.
Temporary free access: https://authors.elsevier.com/c/1iUbN,WNxuZyK
Néstor A. Sánchez-Goycochea & G.- Hernández-Dueñas.Chi
Journal of Mathematical Analysis and Applications (2024)
Link to Journal

 

 

 

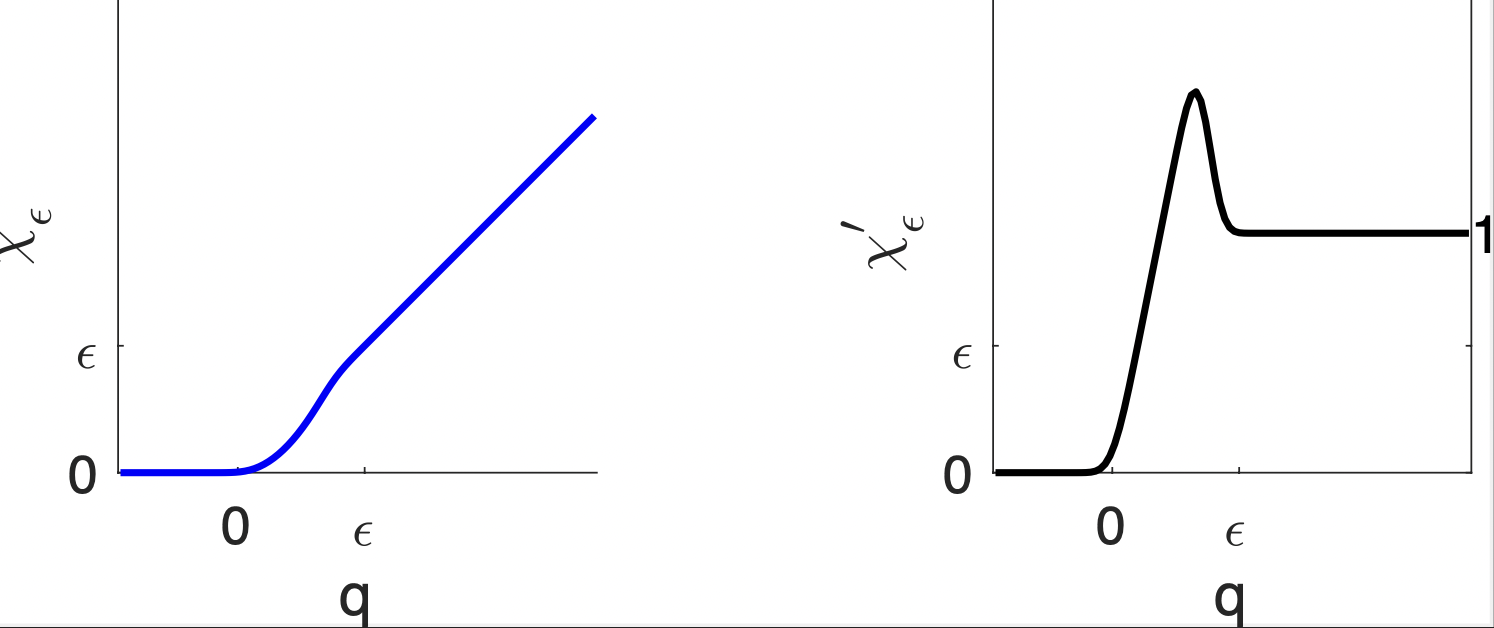
Figure: Graph of transition function from saturated to unsaturated regions.

 
19. A patch in time saves nine: Methods for the identification of localised dynamical behaviour and lifespans of coherent structures.
C. Blachut, C. González-Tokman & G.- Hernández-Dueñas.
Journal of Nonlinear Science (2023)
Link to Journal
 
 
 
 
 
 
 
 
Figure: Identification of coherent structures using dynamical systems
 
18. A new two-dimensional blood flow model with arbitrary cross sections.
C.A. Rosales-Alcantar & G.- Hernández-Dueñas
ESAIM: Mathematical Modeling and Numerical Analysis (2023).
Link to Journal
 
 
 
 
 
 
 
 
 
Figure: Circulation pattern in blood flow simulation
 
 
17. Bathymetry and friction estimation from transient velocity data for one-dimensional shallow water flows in open channels with varying width.  
G. Hernandez-Duenas, M.A. Moreles  & P. Gonzalez-Casanova
Physics of Fluids (2023)
Link to Journal
 
 
 
Figure: Bathymetry estimation with the steepest descent method
 
16. Perturbations of the Landau Hamiltonian: Asymptotics of Eigenvalue Clusters. 
G. Hernandez-Duenas, S. Pérez-Esteva, A. Uribe & C. Villegas-Blass
Ann. Henri Poincaré (2022). https://doi.org/10.1007/s00023-021-01092-7
 
 
 
Link to Journal 
Figure: Diagram of different limiting regimes for the Landau problem
 
 
15. Impact of Wave-Vortical Interactions on Oceanic Submesoscale Lateral Dispersion.
G. Hernandez-Duenas, M. Pascale Lelong, and Leslie M. Smith
Journal of Physical Oceanography (2021).
 
The numerical model simulations upon which this study is based are too large to archive. The velocity and density data at 200 inertial periods as well as the data for the particles' trajectories from 200 to 210 inertial periods are available here.  
 
Link
Figure: Horizontal contours of linear potential vorticity.
 
14. A central-upwind scheme for two-layer shallow-water flows along channels with arbitrary geometry.
G. Hernandez-Duenas & J. Balbas
ESAIM: Mathematical Modeling and Numerical Analysis (2021).
 Link
 
 
 Figure: Schematic of a two layer shallow water flow
 
13. A well-balanced positivity-preserving central-upwind scheme for one-dimensional blood-flow models.
G. Hernandez-Duenas, G. Ramirez-Santiago
International Journal for Numerical Methods in Fluids (2020). https://doi.org/10.1002/fld.4887
 
Link to Journal
 
Figure: Schematic for blood-flow model 
 
 12. Weak- and Strong- Friction Limits of Parcel Models: Comparisons and Stochastic Convective Initiation Time.  G. Hernandez-Duenas, S. Stechmann and L. Smith. Quarterly Journal of the Royal Meteorological Society (2019), Volume 145, No. 722, pp 2272-2291  (2019). 
Link to Journal 

 

Figure: Bistability perspective of conditional stability

 
11. A positivity-preserving central-upwind scheme for isentropic two- phaseflows through deviated pipes.
G. Hernandez-Duenas, Ulises Velasco-García and Jorge Velasco Hernández. ESAIM: Mathematical Modelling and Numerical Analysis (2019). 
Link to Journal 

Figure: Schematic of the two-phase flows in deviated pipes.

 10. A Hybrid Method to Solve Shallow Water Flows with Horizontal Density Gradients.  G. Hernandez-Duenas. Journal of Scientific Computing (2017), Volume 73 (2-3) pp 753-782.
 Link to Journal   
 
 
 
Figure: Radial dam break over flat topography (height, density and pressure)
 
9. A central-upwind scheme with artificial viscosity for shallow-water flows in channels.
G. Hernandez-Duenas and Abdelaziz Beljadid.
Advances in Water Resources 96 (2016) 323-338.
Link to Journal
 
 
 
Figure: Schematic of channel in numerical experiment of dam break.
 
8. Stability and Instability Criteria for Idelized Precipitating Hydrodynamics.
G. Hernandez-Duenas, Leslie M. Smith, and Samuel N. Stechmann.
Journal of Atmospheric Sciences, Vol 72, No. 6 (2015), pp. 2379-2393
Link to Journal
 
 
Figure: Growth rates versus horizontal wavenumbers for different values of rainfall speed. 
 
7. Algebras of semiclassical pseudodifferential operators associated with Zoll-type domains in cotangent bundles
G. Hernández-Dueñas and Alejandro Uribe.
Journal of Functional Analysis, 268 no. 7 (2015), pp. 1755-1807
Link to Journal
 
 
 
Figure: Propagation of a coherent state in a Zoll-type domain.
 
6. A Positivity Preserving Central Scheme for Shallow Water Flows in Channels with Wet-Dry States.
Jorge Balbás and G. Hernández-Dueñas. 
ESIAM: Mathematical Modelling and Numerical Analysis (M2AN) 48 (2014) 665-696.
Link to Journal
 
Figure: Dam break simulation at different times.
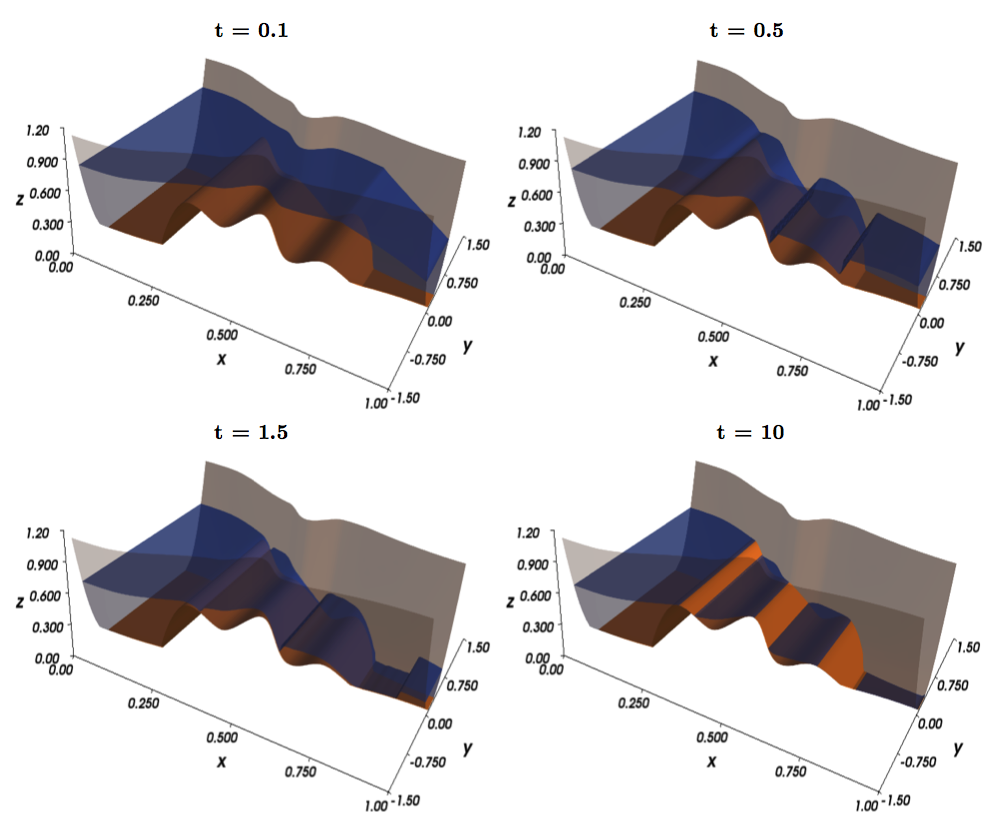
Blue: Water height. Brown: Bottom topography. Gray: Walls.
 
 
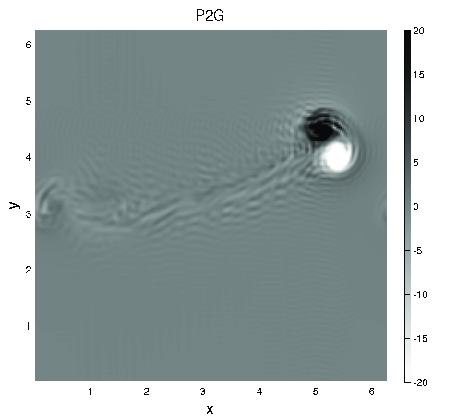
5. Investigation of Boussinesq dynamics using intermediate models based on wave-vortical interactions.
G. Hernandez-Duenas, Leslie M. Smith, and Samuel N. Stechmann
Journal of Fluid Mechanics, 747 (2014), 247-287
Link to Journal
 
 
 
 Figure: Dipole evolution given by the Boussinesq model
 
4.  Minimal models for precipitating turbulent convection
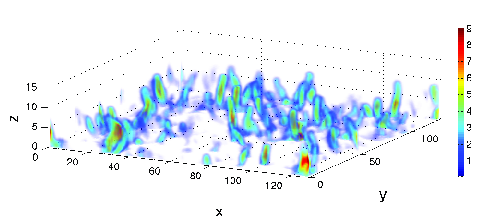
G. Hernandez-Duenas, Andrew J. Majda, Leslie M. Smith, and Samuel N. Stechmann.
Journal of Fluid Mechanics, 717 (2013), 576-611.
 
Link to Journal
 
 
 
Figure: Contours of rain water. Scattered convection (left) versus squall lines (right).
 
3. Shallow Water Flows in Channels.
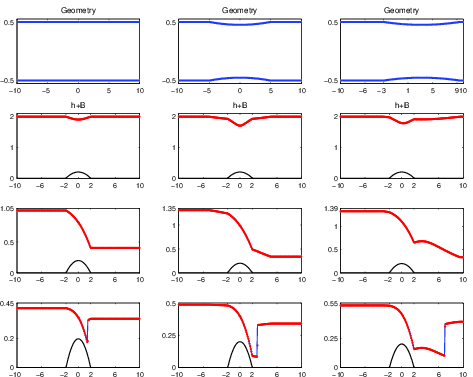
G. Hernández and Smadar Karni.
J. Sci. Comput. 48 (2011), no. 1-3, 190-208.
Link to Journal
 
 
Figure: Exact and numerical steady state (discontinuous transcritical) solutions to shallow water.
 
2. A Hybrid Algorithm for the Baer-Nunziato Model Using the Riemann Invariants.
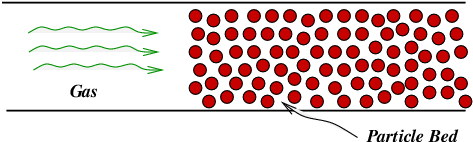
Smadar Karni and G. Hernández-Dueñas.
J Sci Comput, 45, (2010), 382-403.
 
Link to Journal
Figure 1: Schematic: Gas flow over a porous particle bed.

1.A Hybrid Scheme for Flows in Porous Media.
Smadar Karni and G. Hernández-Dueñas.
Hyperbolic Problems: Theory, Numerics, Applications.
Proceedings of Simposia in Applied Mathematics, Volume 67, Part 2, (2009), 715-724.
Amer. Math. Soc., Providence, RI, (2009).

Link to Journal

Submitted papers:

 1.  S. Shah & G.- Hernandez-Duenas. A weakly-compressible two-layer shallow water model in general channels.
 
2. O. Guerrero-Medina & G. Hernandez-Dueñas. Exploring the excess of cloud condensation nuclei and rain suppression using a minimal 3D Boussinesq model with bulk cloud microphysics.

Papers in preparation:

1. Jeffrey J. Early, Gerardo Hernández-Dueñas, Leslie M. Smith & M.-Pascale Lelong. Exact expressions for available potential energy and available potential vorticity.

Extended abstracts:

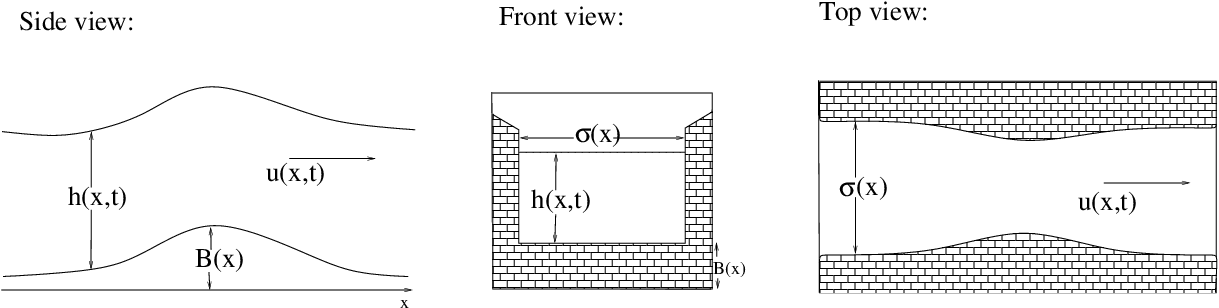
A Scheme for Shallow Water Flow with Area Variation.
Smadar Karni and G. Hernández-Dueñas.
American Institute of Physics.
AIP Conference Proceedings
International Conference on Numerical Analysis and Applied Mathematics
Rethymno, Crete, Greece, 18-22 September 2009. 1168 (2009), 1433-1436.

Link to Journal